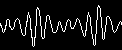Ihypress Multimedia ⛯ Sine Waves and Musical Tones

Combining Sine Waves to Produce Musical Tones and the Human Voice

Sounds can be broken up into sine waves (see picture below) that have different frequencies and amplitudes. These sine waves can be played at the same time to recreate the original sound.

Any two notes on the left-hand side that are played at the same time should create a pleasant sound (consonance) because of the simple ratios. Any note on the right-hand side played with any of the other notes should give a less pleasant sound (dissonance) and demonstrates "beats". Click on the "PLAY" button for each note and the "PAUSE/STOP" button when you are done.

200 Hz	210 Hz
300 Hz	220 Hz
400 Hz	230 Hz
500 Hz	240 Hz
600 Hz	290 Hz

The 300 Hz and 290 Hz sounds create the strongest dissonance because they are the closest to each other, but not close enough to be interpreted as the same sound. You can hear the "beats" in this combination, which are occuring 10 times per second, which is the difference between the two frequencies.

Musical Instrument Sound Qualities

Each instrument has frequencies in addition to the fundamental frequency that combine to create the instrument's distinctive sound. The smooth sound on some instruments (e.g. the flute) is caused by the simple ratios of those frequencies. Harsher sounding instruments consist of frequencies whose ratios are more complex.

Flute

The following three sine waves should approximate the sound of a flute playing "A" at 220 Hz. The flute is not as accurate as the other simulations that follow. The number after the Hz number is the amplitude of the sine wave. The resultant waveform from the combination of frequencies is also shown.

220 Hz / 100

440 Hz / 10

660 Hz / 40

Violin

The following simple sine waves create the sound of a violin playing "G", 196 Hz, on the open fourth string. It is adjusted to 200 Hz so that the ratios could be seen more easily. Note that the violin does not have a 200 Hz signal component when playing the note corresponding to 200 Hz.

200 Hz / 76	600 Hz / 100
800 Hz / 44	1000 Hz / 44
1200 Hz / 32	1600 Hz / 32
3400 Hz / 16

The Human Voice

Some vowels are easy to simulate. Here are the vowel sounds ma, maw, mow, and mooo. Notice the decrease in frequency. "Sing along" to hear some dissonance. ;)

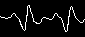
ma: 910 Hz	maw: 732 Hz
mow: 460 Hz	moo: 326 Hz

Other vowels require two frequencies. Here are the vowels in mat, met, mate, and meet. Notice that the higher frequency has much less amplitude. These two frequencies of each vowel are generated by the mouth and pharynx air chambers which resonate in response to air passing through the vocal cords. Since the mouth is smaller than the pharynx, it creates the higher frequency in each syllable (right hand column). For example, compare how far below the roof of your mouth your tongue is when saying the vowel in "mat" (1,840 Hz) compared to how close it is to the roof (creating a smaller chamber) when saying the vowel in "meet" (3,100 Hz).

	Sound caused by pharynx	Sound caused by mouth chamber
Mat	800 Hz / 100	1840 Hz / 5
Met	690 Hz / 100	1950 Hz / 5
Mate	490 Hz / 100	2460 Hz / 7
Meet	310 Hz / 100	3100 Hz / 5

The following should re-create a saprano singing "ah" as in "father".

310 Hz / 36	1240 Hz / 34
620 Hz / 30	1550 Hz / 27
930 Hz / 100

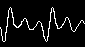
Here is the same vowel "ah" by a bass singer. The dominant frequency in both singers is 930. This is close to the 910 signal seen in "ma" above. The frequencies for syllables do not have to be exact.

310 Hz / 21	1085 Hz / 32
775 Hz / 43	1240 Hz / 32
930 Hz / 100

Other Sound Tidbits

Humans can hear from about 25 to 20,000 Hz. Hertz (Hz) is the number of repeating and identical cycles of sound pressure waves in the air per second. Middle A is 440 Hz and the other notes on a keyboard (for example) are determined by the equation 440*2^(N/12) where "N" is a whole number representing the number of notes up (positive whole number) or down (negative whole number) from middle A. Middle C is 261.6 Hz. The black keys on a keyboard are treated the same as white keys in this equation. The white keys are differentiated from the black keys because the white keys consist of the simplest ratios to each other. This equation creates a "tempered" scale which means that the ratios between the various frequencies are not exactly the simple ratios that are desired because of the effort to try and get all the possible simple ratios on a repeating scale. 440 Hz started to become the standard "A" for instruments around the year 1896.

Credits

Adapted from the original page made by Scott Roberts.